Have you ever wondered why a bridge can suddenly start shaking violently or why a wine glass shatters when a singer hits a high note? This blog explores the fascinating concepts of natural frequency and resonance frequency, revealing how they impact everything from engineering marvels to everyday objects. Expect to uncover the secrets behind these vibrations and learn how they shape our world.
In daily analysis, we often confuse natural frequency and resonance frequency, and think that they are the same thing.
In fact, this is not rigorous.
Natural frequency is the performance of structural natural characteristics, while resonance frequency is the performance of structural response under external forces.
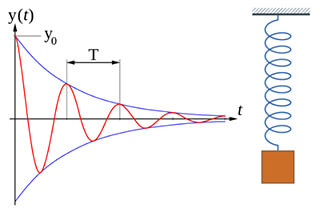
A single degree of freedom system is a system where the position can be fully determined by only one generalized coordinate at any given time. In simpler terms, the force acting on a body is in only one direction. The motion of the doll in the figure below can be represented as a spring-mass system.
A simplified model of the spring mass system can be shown in the following figure.
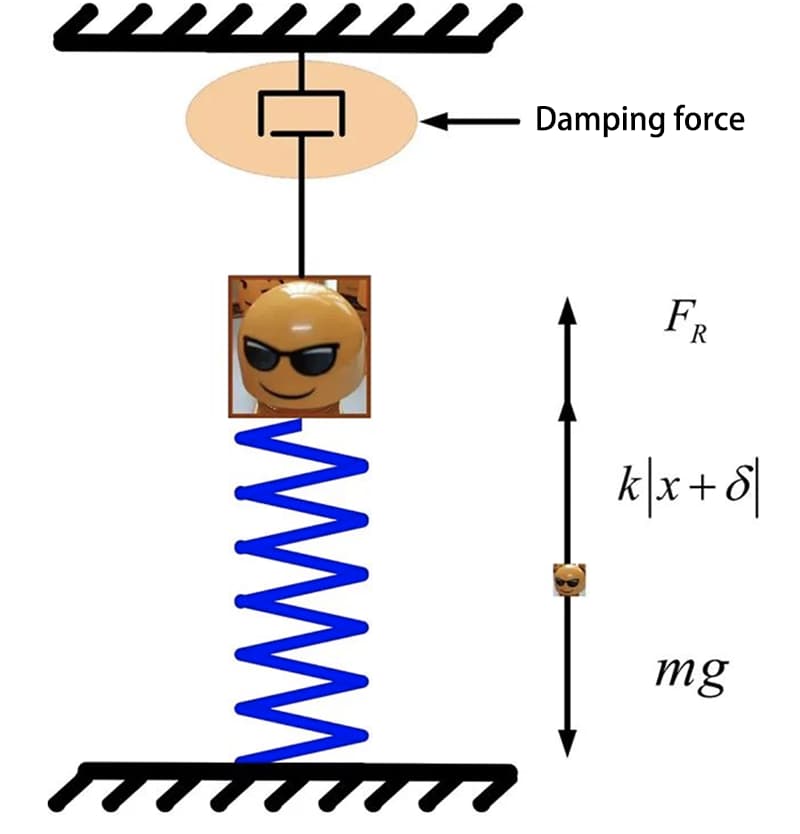
The static balance position of the pickup block is considered the coordinate origin, and it is considered positive when it moves vertically downward along the direction of spring deformation. The distance between the block and the balance position can be represented as x, and the differential equation of motion for the block can be expressed as:
Where, m is the mass of the block, k is the spring stiffness, c is the viscosity coefficient, 2n=c/m is the damping attenuation coefficient, and when the damping coefficient is zero, it corresponds to the undamped vibration system.
Natural frequency Pn:
The natural frequency is only dependent on mass and stiffness, and is not affected by factors such as damping. Structural boundary connections, material properties, shape, and other factors may impact the natural frequency, but these influences are reflected in stiffness and mass and are not the ultimate determining factors.
Free vibration is the vibration of the system without external excitation, and the motion track is related to the initial state and natural characteristics.
The forced vibration refers to the vibration generated by the system under external excitation.
The external excitation is generally a periodic or aperiodic function of time, among which the simple harmonic excitation is the simplest.
Let the simple harmonic exciting force be:
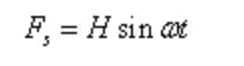
Where, H is the amplitude of exciting force, ω is the angular frequency of the exciting force.
When the block deviates from the equilibrium position by x distance, the differential equation of motion of the block is
Where, h=H/m, the above equation is the differential equation of forced vibration of single degree of freedom with viscous damping, which is a second-order constant coefficient linear non-homogeneous ordinary differential equation.
The above equation is completely consistent with the capacitive load voltage response expression we have learned in circuit theory, which is a second-order constant coefficient linear non-homogeneous ordinary differential equation.
The damping in the circuit depends on the resistance, because the resistance only consumes and does not store energy.
Under simple harmonic excitation, the total solution of the differential equation of motion of a damped system is set as:
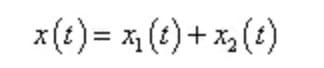
Where, x1 (t) is a homogeneous solution, which is free attenuated vibration;
Due to the existence of damping, the damped vibration part will disappear after a certain period of time.
Its solution is the same as that of free vibration, so it will not be repeated here.
Here we are concerned about the special solution x2 (t) generated by forced vibration, which can be expressed as:
Among them,
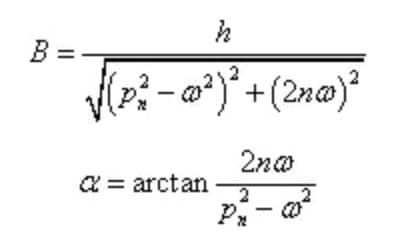
The amplitude and phase lag of the steady-state forced vibration are independent of the initial conditions, but only depend on the characteristics of the system and the excitation.
Set the frequency ratio, damping ratio and amplitude amplification coefficient as:
The above formula can be rewritten as:
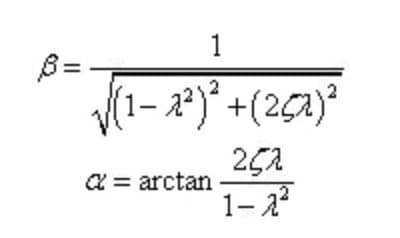
Based on this, we can know the response of the system under the forced vibration.
For better understanding, the relationship between different damping ratios, frequency ratios, and amplitude amplification coefficients can be illustrated. The frequency is separated into three regions: low-frequency, medium-frequency, and high-frequency. As seen in the figure, in both the low-frequency and high-frequency areas, the damping has a minimal impact on the amplitude amplification coefficient. Thus, the damped system can be simplified as an undamped system for easier calculation.
The natural frequency is an inherent characteristic of a system’s structural properties, determined solely by its mass and stiffness, independent of external factors such as damping. In an n-degree-of-freedom system, there exist n distinct natural frequencies, while continuous systems possess an infinite number of natural frequencies.
Resonance occurs when an external excitation frequency closely matches or coincides with a system’s natural frequency, causing the structure to vibrate with significantly increased amplitude. This phenomenon is represented by the blue circle in the accompanying figure. The resonant frequency, while often approximating the natural frequency in lightly damped systems, can deviate from it in heavily damped structures, typically being lower than the natural frequency.
In system design, it is crucial to avoid not only the specific resonant peak frequency but also the surrounding resonant band, corresponding to the intermediate frequency region in the figure. This precaution is necessary because the system’s response remains considerably amplified within this band. Engineers must ensure that neither the system’s normal operation nor external excitations from nearby equipment fall within this critical range.
Resonance can have profound implications, both beneficial and detrimental. Negative effects may include the collapse of lifting beams, ground resonance in helicopters, machinery damage, and infrasonic-induced structural failures. Conversely, resonance is deliberately utilized in musical instruments to produce desired tones and harmonics.
To mitigate risks associated with resonance, designers employ various strategies:
Understanding and managing resonance is essential in fields ranging from civil engineering and aerospace to precision manufacturing and musical instrument design. Advanced analytical tools, such as finite element analysis and experimental modal analysis, play crucial roles in predicting and characterizing resonant behavior in complex systems.
As the founder of MachineMFG, I have dedicated over a decade of my career to the metalworking industry. My extensive experience has allowed me to become an expert in the fields of sheet metal fabrication, machining, mechanical engineering, and machine tools for metals. I am constantly thinking, reading, and writing about these subjects, constantly striving to stay at the forefront of my field. Let my knowledge and expertise be an asset to your business.
EN 
AR 
DE 
ES 
FR 
IT 
JA 
NL 
PT 
PT_BR 
RU 
KO