In this article, we explore the fascinating concepts of strength and stiffness in engineering. You’ll learn how these principles ensure the safety and durability of everyday objects, from bridges to smartphone screens. Join us to uncover the secrets behind engineering marvels!
To ensure the optimal performance and reliability of a mechanical system or structure, each component must fulfill its intended function effectively and efficiently. The primary objective of engineering component safety design is to guarantee that all elements possess adequate strength, stiffness, and stability, thereby ensuring the overall integrity and longevity of the system.
Stability is a fundamental concept in engineering, referring to a component’s ability to maintain or regain its original equilibrium state when subjected to external forces. This principle is critical in various scenarios, such as:
These examples illustrate the importance of stability in preventing catastrophic failures and ensuring the safe operation of mechanical systems and structures.
In this discussion, I will focus on elucidating my comprehensive understanding of two equally crucial engineering principles: stiffness and strength. These concepts, along with stability, form the triad of fundamental considerations in component safety design, each playing a vital role in determining the overall performance, durability, and safety of engineered systems.
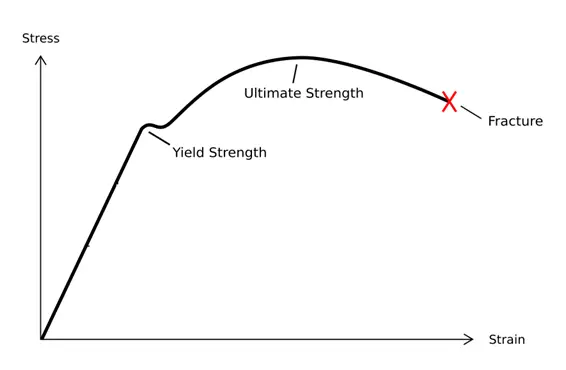
Definition: Strength is the capacity of materials, components, or structures to withstand applied loads or forces without failure, excessive deformation, or damage. It is a fundamental property in materials science and engineering that determines the ability of a material to resist mechanical stress.
Strength encompasses various aspects, including:
For example, in metal fabrication, understanding the strength of materials is crucial for designing components that can withstand operational loads. A steel beam in a building must have sufficient strength to support the structure’s weight and additional loads without bending or breaking.
Strength is influenced by various factors, including:
The unit of measurement for strength is typically expressed in megapascals (MPa) or pounds per square inch (psi) in imperial units. For instance, mild steel has a tensile strength of approximately 400-550 MPa, while high-strength alloy steels can exceed 1000 MPa.
In manufacturing and engineering, strength calculations and testing are essential for:
Advanced techniques such as finite element analysis (FEA) and destructive testing are often employed to assess and validate the strength of materials and components in complex engineering applications.
Brittle fracture: A sudden and catastrophic failure mode characterized by rapid crack propagation with minimal plastic deformation. This type of failure occurs without warning and typically results in a clean, flat fracture surface.
For example:
Plastic yield: A failure mode where the material undergoes significant plastic deformation, resulting in permanent shape change and loss of structural integrity. This type of failure is generally more gradual and observable compared to brittle fracture.
For example:
It’s important to note that the failure mode of a material depends on various factors, including material properties, loading conditions, temperature, and environmental factors. Some materials may exhibit a transition from ductile to brittle behavior under certain conditions, such as low temperatures or high strain rates.
1. Maximum tensile stress theory:
When the maximum tensile stress σ1 at a point in the member reaches the ultimate stress σb under unidirectional stress conditions, the material will undergo brittle fracture. Thus, the criteria for brittle fracture failure of components with critical points under complex stress conditions are: σ1 = σb.
Consequently, the strength conditions established by the first strength theory are: σ1 ≤ σb.
2. Maximum tensile strain theory:
When the maximum tensile strain ε1 reaches the limit value εu under unidirectional stress conditions, the material will fail due to brittle fracture. This can be expressed as ε1 = εu.
From the generalized Hooke’s Law, we can calculate ε1 as: ε1 = [σ1 – u(σ2 + σ3)] / E, so σ1 – u(σ2 + σ3) = σb.
The strength conditions established by the second strength theory are: σ1 – u(σ2 + σ3) ≤ σb.
3. Maximum shear stress theory:
When the maximum shear stress τMax reaches the ultimate shear stress τ0 under unidirectional stress conditions, the material will fail due to yielding. This can be expressed as τMax = τ0.
The formula for the shear stress on an inclined section during axial tension is τ0 = σs/2 (σs being the normal stress on the cross-section). The formula for τMax is (σ1 – σ3)/2. Thus, the damage condition can be rewritten as σ1 – σ3 = σs.
The strength condition established by the third strength theory is: σ1 – σ3 ≤ σs.
4. Shape change specific energy theory:
When the shape change ratio at a point in the member reaches the limit value under unidirectional stress conditions, the material will fail due to yielding.
The strength condition established by the fourth strength theory is:
√(σ1^2 + σ2^2 + σ3^2 – σ1σ2 – σ2σ3 – σ3σ1) < σs.
Definition: Stiffness refers to the ability of a material, component, or structure to resist elastic deformation or displacement when subjected to an external force. It quantifies the extent to which a system can maintain its shape and dimensions within allowable limits under applied loads.
Stiffness is a fundamental parameter in engineering that characterizes the relationship between force and deformation in a material or structure. It is defined as the ratio of applied force to the resulting displacement, indicating the amount of force required to produce a unit of deformation. In mathematical terms, stiffness (k) is expressed as:
k = F / δ
Where:
The unit of stiffness is typically force per unit length, such as N/m (Newtons per meter) in the SI system or lbf/in (pounds-force per inch) in the imperial system.
In practical terms, stiffness can be conceptualized using the analogy of a spring. The spring constant, which represents the stiffness of a spring, is defined as the ratio of the applied tensile or compressive force to the resulting elongation or compression. This relationship is described by Hooke’s Law for linear elastic systems.
Understanding and controlling stiffness is crucial in various engineering applications, including:
Engineers must balance stiffness requirements with other design considerations such as strength, weight, cost, and manufacturability to create optimal solutions for specific applications.
When the applied load is constant, it is referred to as static stiffness.
When the load alternates, it is called dynamic stiffness.
Static stiffness encompasses structural stiffness and contact stiffness.
Structural stiffness refers to the stiffness of the member itself and includes bending stiffness and torsional stiffness.
1. Bending stiffness: calculated according to the following formula:
K=P/δ
Where
2. The torsional stiffness is calculated according to the following formula:
Km=ML/θ
Where M — applied torque (n · m);
L — distance from the torque action position to the fixed end (m);
θ—— Torsion angle (°)
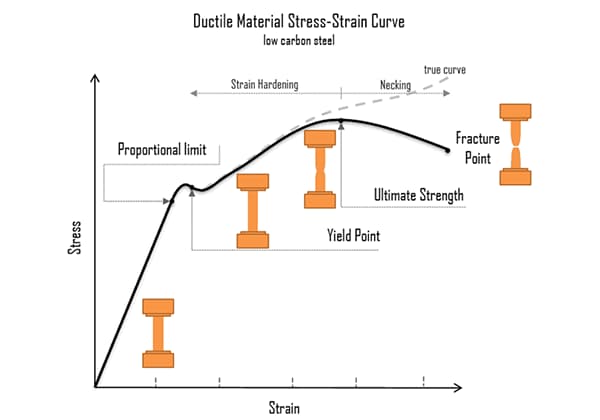
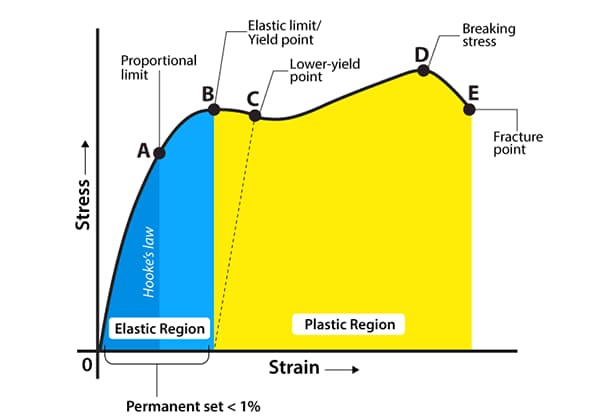
From the above explanation of strength and stiffness, it can be seen that strength focuses on failure under external force and is classified into plastic yield and brittle fracture failure, which is related to the stress-strain curve during tensile testing. In comparison, stiffness pertains to the relationship between deformation and force.
As shown in the fig.
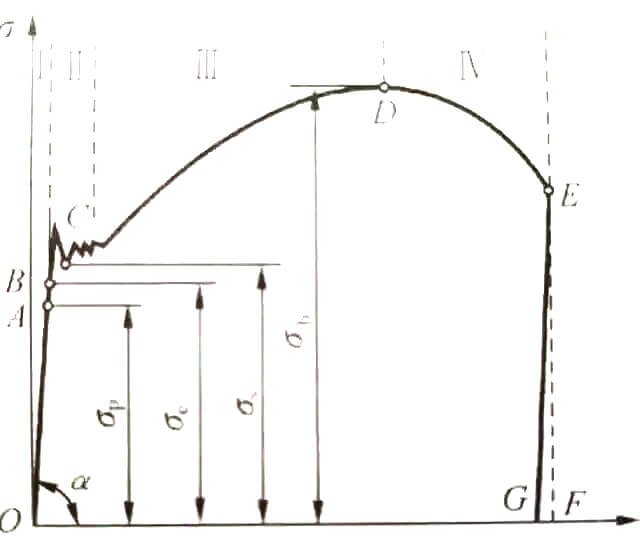
The curve in the figure can be divided into four stages:
1. Elastic deformation stage;
2. Yield stage;
3. Strengthening stage;
4. Local necking stage.
Stiffness is defined as resistance to elastic deformation, which occurs in the initial stage, and is governed by Hooke’s law under elastic conditions.
Calculating the bending stiffness and torsional stiffness under static loads is similar to Hooke’s law, suggesting that stiffness is only measured during the elastic deformation stage.
In the next stage, when plastic deformation occurs during tensile testing, the residual strain does not disappear. On the stress-strain curve, although stress remains almost unchanged, the strain increases significantly. At this point, the stress reaches the yield limit and the material enters the stage of plastic yield failure. As the stress continues to increase, the strain also increases until it reaches the strength limit.
Therefore, the measurement of strength occurs after the material undergoes elastic deformation and before it reaches the strength limit.
In conclusion, both stiffness and strength are evaluated during the failure stage of parts, with stiffness being measured by stress and strength being measured by deformation.
In terms of their order in the deformation process, stiffness occurs in the earlier stage while strength occurs in the later stage.
Hence, in evaluating the failure conditions of parts, as long as the stiffness requirements are met, the part should be able to withstand sufficient stress during the elastic deformation stage, which in turn should meet the strength requirements.
This relationship is reflected in various designs, such as the shaft in mechanical equipment. Typically, the shaft size is determined based on strength conditions, and then its stiffness is verified based on stiffness conditions.
Therefore, the stiffness requirements for precision machinery shafts are set very high, and the design of its cross-sectional size is often controlled by stiffness conditions.
As the founder of MachineMFG, I have dedicated over a decade of my career to the metalworking industry. My extensive experience has allowed me to become an expert in the fields of sheet metal fabrication, machining, mechanical engineering, and machine tools for metals. I am constantly thinking, reading, and writing about these subjects, constantly striving to stay at the forefront of my field. Let my knowledge and expertise be an asset to your business.
EN 
AR 
DE 
ES 
FR 
IT 
JA 
NL 
PT 
PT_BR 
RU 
KO